The interquartile range is the difference between quartile 3 (upper quartile) and quartile 1 (lower quartile). It is one way of describing the spread of the data.



Let's look at a couple of examples.

Find the interquartile range of the following data.

Example 1:

1, 7, 0, 7, 2, 6, 3, 6, 0, 7, 8

First make sure that it is in order from least to greatest.

0, 0, 1, 2, 3, 6, 6, 7, 7, 7, 8

Find the median:0, 0, 1, 2, 3, 6, 6, 7, 7, 7, 8  6 is the median (middle number and Q₂)

Find the middle of the 0, 0, 1, 2, 3, 6, 6, 7, 7, 7, 8  Q₁ = 1 first half of the numbers

Find the middle of the 0, 0, 1, 2, 3, 6, 6, 7, 7, 7, 8  Q₃= 7Second half of the numbers



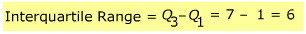
Example 2: 10, 1, 7, 5, 1, 8, 5, 4, 6, 5, 9, 12

Put in order from least to greatest

1, 1, 4, 5, 5, 5, 6, 7, 8, 9, 10, 12

find the average

Find the median   1, 1, 4, 5, 5, 5, 6, 7, 8, 9, 10, 12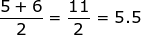   

Find Q₁ the median of the lower half

1, 1, 4, 5, 5, 5, 6, 7, 8, 9, 10, 12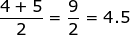  

Find Q₃the median of the upper half

1, 1, 4, 5, 5, 5, 6, 7, 8, 9, 10, 12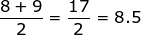  



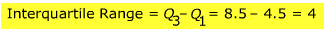
Let's look at an example when given a box and whisker plot.


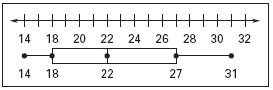
Min         Q₁           Med         Q₃         Max



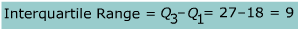
A quick review: to find the interquartile range you will put the data in order from least to greatest then find the median. Once you have found the median Q₁is the median of the first half of the data and Q₃is the median of the second half of the data.

Related Links: Math algebra Measures of Variability : Range Parts of An Expression