The Mean Value Theorem
MEAN VALUE THEOREM
Conditions:
2. The function f must be differentiable on the open interval (a, b).
If these two conditions are met then there is a number c in the open interval (a, b) such that:
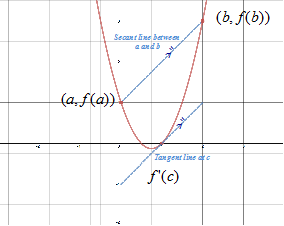
Let's use this theorem in a couple examples.
|
Step 1: Determine if the Mean Value Theorem can be applied. |
To apply the Mean Value Theorem the function must be continuous on the closed interval and differentiable on the open interval. This function is a polynomial function, which is both continuous and differentiable on the entire real number line and thus meets these conditions. |
|
Step 2: Find the slope of the secant line. |
Determine f(a) if a = -1, the left endpoint of the interval.
Determine f(b) if b = 1, the right endpoint of the interval.
Determine the slope of the secant line between -1 and 1.
|
|
Step 3: Determine the first derivative of the function. |
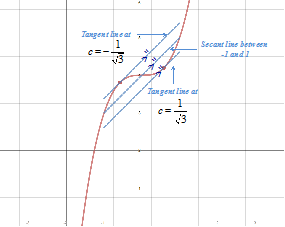
f(x) = x3 + 4
|
|
Step 4: Set and solve for c. Mean Value Theorem Sub. from Step 2 3c2 = 1 Sub. for Divide by 3 Square root and
There are two values of c in the interval of [-1, 1] for which the tangent line of c is parallel to the secant line between -1 and 1. |
|
|
Step 1: Determine if the Mean Value Theorem can be applied. |
To apply the Mean Value Theorem the function must be continuous on the closed interval and differentiable on the open interval. This function is a rational function, which is both continuous on the interval [1, 3]and differentiable on the interval (1, 3). |
|
Step 2: Find the slope of the secant line. |
Determine f(a) if a = 1, the left endpoint of the interval.
Determine f(b) if b = 3, the right endpoint of the interval.
Determine the slope of the secant line.
|
|
Step 3: Determine the first derivative of the function. |
|
|
Step 4: Set and solve for c. Mean Value Theorem Sub. from Step 2 Sub. for Divide by -3 c2 = 3 Take reciprocal Square root and (but this point is not in the interval)
There is only one value for c in the interval of [1, 3] for which the tangent line of c is parallel to the secant line between 1 and 3. |
|
|
Related Links: Math algebra Limits: Introduction and One-Sided Limits Limits: Infinite Limits Calculus Topics |
To link to this The Mean Value Theorem page, copy the following code to your site: