Rolle's Theorem

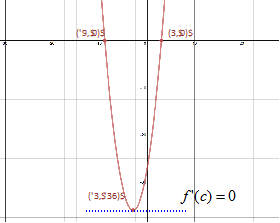
Step 1: Find the x-intercepts or zeros of the function.

x² + 6x - 27 = 0 Original

(x + 9)(x - 3) = 0 Factor

$x+9=0\to x=-9$    Set to zero & solve

$x-3=0\to x=3$      Set to zero & solve

Step 2: Find the first derivative of the function.

$f\left(x\right)=x^2+6x-27$

$f\prime \left(x\right)=2x+6$

Step 3: Solve for $f^{\prime }\left(x\right)=0$.

0 = 2x + 6 $f^{\prime }\left(x\right)=0$

-3 = x Solve

Step 4: Compare x-intercepts to the value in Step 3 when $f^{\prime }\left(x\right)=0$.

$f^{\prime }(-3)=0$ and -3 falls between -9 and 3, therefore there is a minimum point at (-3, -36).

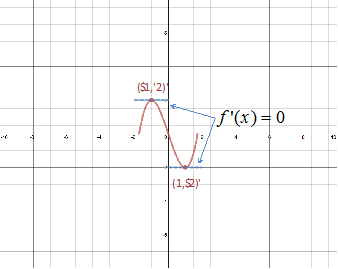
Step 1: Find the first derivative of the function.

f(x) = x³ - 3x

$f^{\prime }\left(x\right)=3x^2-3$

Step 2: Set the first derivative equal to zero and solve.

$f^{\prime }\left(x\right)=3x^2-3$ First derivative

0 = 3x² - 3      Set $f^{\prime }\left(x\right)=0$

0 = 3(x² - 1)   Factor out a 3

0 = 3(x - 1)(x + 1)    Factor the quad.

$x-1=0\to x=1$    Solve for x

$x+1=0\to x=-1$   Solve for x

Step 3: Substitute the x-coordinates found in Step 2 into the function to determine the corresponding y-coordinates.

$f\left(1\right)={\left(1\right)}^3-3\left(1\right)=-2$   f(1) = -2

$f\left(-1\right)={\left(-1\right)}^3-3\left(-1\right)=3$   f(-1) = 2

Step 4: Name the coordinates

The coordinates of the two points where $f\prime \left(x\right)=0$ are (1, -2) and (-1, 2).