Orbital speed Formula
In gravitationally linked systems, the orbital speed of a body or astronomical object is the speed at which it orbits around the barycenter or, if the object is much less massive than the largest body in the system, its relative velocity to that larger body. The speed in the latter case may be relative to the surface of the largest body or relative to its center of mass.
The term can be used to refer to the mean orbital speed, the mean velocity in an entire orbit, or its instantaneous speed at a given point in its orbit. The maximum orbital velocity (instantaneous) occurs in the periapsis, while the minimum speed for objects in closed orbits occurs in the apogee. In ideal two-body systems, objects in open orbits continue to slow down forever as their distance to the centre of gravity increases.
orbital speed = square root (gravitational constant * mass of the attractive body / radius of the orbit)
The equation is:
,
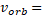
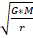We have:
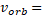orbital speed.
G = the gravitational constant.
M = mass of the attractive body.
r = radius of the orbit.
Orbital speed Questions:
1) What is the speed orbital of the earth?
Answer: First, we look for default values for the earth, such as mass and its approximate radius.
M = 5.98*1024 kg.
G = 6.67*10-11Nm2/kg2.
r = 6370km.
= 
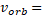
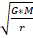= 7,913.05m/s.
vorb = 7,913.05m/s.
2)The International Space Station describes a circular orbit around the Earth some 400 km above it. Applying the law of universal gravitation and Newton's second law to its centripetal acceleration, it calculates the space station's orbital velocity in km/h.
Answer: The radius of the Earth is 6371 km, so the distance at which the space station is located from the center of the Earth will be 6771 km (adding the 400 km height).
M = 5.98*1024 kg.
G = 6.67*10-11Nm2/kg2.
r = 6771km.
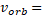
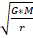= = 7,675.15m/s.
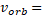7,675.15m/s.
|
Related Links: |